Neuromorphic Time Crystal Networks: Polariton Exciton Condensate as an Analog to String Time Crystal
The concept of neuromorphic computing has become increasingly prominent as we push the boundaries of unconventional computation. One particularly exciting avenue lies in exploring time crystal networks based on exciton-polariton condensates—systems that straddle the worlds of photonic and electronic excitations. These hybrid quasiparticles, when treated under specific conditions, exhibit emergent behaviors that are analogous to those of string time crystals, providing new avenues for advancing computation and network dynamics.
Exciton-polariton condensates are formed through strong coupling between photons and excitons within semiconductor microcavities (Carraro Haddad et al., 2024). In such settings, they exhibit macroscopic quantum coherence and spontaneously organize into time crystal phases—a state of matter that breaks time translational symmetry. Recent research has demonstrated that exciton-polariton condensates, trapped within microcavities and subjected to continuous-wave optical pumping, display behaviors akin to those of both continuous and discrete time crystals. This is achieved via non-equilibrium phase transitions, where condensates oscillate spontaneously without external periodic driving, essentially embodying a time-crystalline state within a non-equilibrium framework (Carraro Haddad et al., 2024).
A particularly intriguing analogy is found when exciton-polariton condensates are compared to string time crystals, an exotic quantum state characterized by long-lived oscillations akin to periodic string vibrations. In polariton lattices, interactions between polaritons lead to bifurcations in spin states, spontaneous ferromagnetization, and the emergence of stable collective modes that evolve over time. These temporal oscillations mirror the periodic nature of a vibrating string, wherein the condensate phases represent analogous modes of oscillation (Cheng et al., 2024; Martins et al., 2023).
Moreover, time crystal behavior in exciton-polariton condensates is closely related to the interplay between phonon-exciton interactions and externally applied potentials. In systems such as transition metal dichalcogenide (TMDC) strips or microcavity traps, polaritons exhibit semiclassical time crystal properties. This is particularly evident when considering the strong coupling effects that enhance the coherence of the condensate, which serves as a built-in mechanism to sustain periodic oscillations across the lattice, much like a classical time crystal that follows its own internal clock (Martins et al., 2023; Nalitov et al., 2019).
Beyond their analogy to string time crystals, these polariton systems offer exciting opportunities for neuromorphic computing. Polariton-based neuromorphic networks leverage the unique properties of exciton-polaritons, such as their strong nonlinearity and rapid response times, to achieve highly efficient processing in spiking neural networks (SNNs). The leaky integrate-and-fire mechanism in exciton-polariton condensates enables photonic spiking neuron behavior, which is employed for unconventional computational tasks. The intrinsic ability of polaritons to behave like biological neurons offers a promising route towards energy-efficient, brain-like computational architectures (Tyszka et al., 2022; Opala & Matuszewski, 2023).
The potential applications of neuromorphic time crystal networks extend beyond conventional digital computing. Due to the unique properties of exciton-polaritons, these systems are instrumental in solving complex optimization problems, performing tasks that require high parallelism, and even functioning as quantum simulators. The combination of optical nonlinearity, rapid response times, and strong coherence makes exciton-polariton condensates ideal candidates for implementing unconventional computing paradigms that go beyond the von Neumann architecture. For instance, their application in reservoir computing—a framework that uses dynamic systems as computational reservoirs—has shown promising results in pattern recognition tasks, significantly outperforming traditional approaches in terms of energy efficiency and processing speed (Sedov & Kavokin, 2024).
Additionally, polariton-based systems help bridge the gap between classical and quantum computing. By utilizing the quantum properties of polaritons, such as superposition and entanglement, these systems willl pave the way for hybrid classical-quantum computing platforms. Such platforms would leverage the best of both worlds—quantum coherence for solving inherently quantum problems and classical neuromorphic architectures for tasks requiring massive parallelism and real-time processing.
In conclusion, exciton-polariton condensates not only serve as a platform for realizing neuromorphic time crystal networks but also embody the features of string time crystals through their coherent, periodic behavior. By blending the knowledge of polariton exciton dynamics and string time crystals, we explore new paradigms of computation and network organization, ultimately advancing both fundamental understanding and technological applications of quantum-inspired computing systems. The future of neuromorphic time crystal networks holds immense promise, with potential breakthroughs in computational efficiency, quantum-classical hybrid systems, and novel architectures for tackling some of the most challenging problems in science and technology.
Synaptic Transistor and Neuromorphic Time Crystal
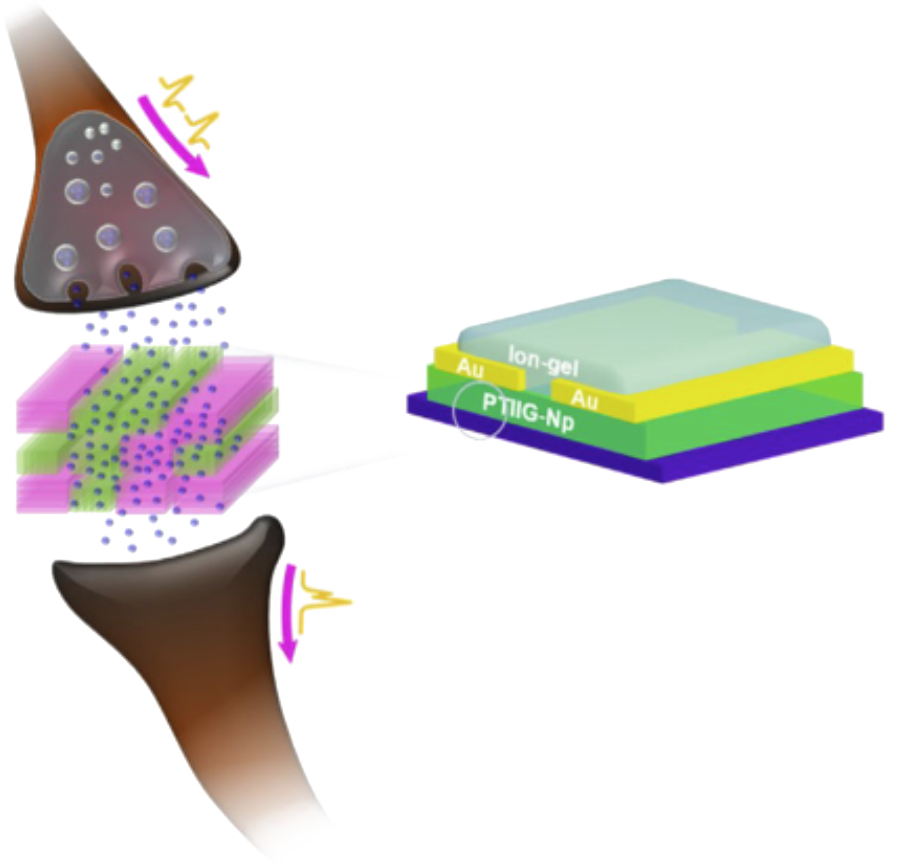
Synaptic transistors play a crucial role in bridging the gap between traditional neuromorphic systems and novel computing paradigms like neuromorphic time crystals. A synaptic transistor is an electronic device that mimics the behavior of biological synapses, acting as a connection point between artificial neurons that can regulate the flow of information in response to varying stimuli, much like real synapses in the human brain. These devices are essential for enabling adaptive learning, dynamic plasticity, and efficient parallel processing, key attributes of neuromorphic computing.
When used in conjunction with neuromorphic time crystal networks, synaptic transistors can provide additional levels of control and programmability. The exciton-polariton condensates in time crystal systems already possess properties that mimic neural spiking behavior, but incorporating synaptic transistors offers a more sophisticated mechanism for modifying the strength and timing of synaptic connections. This allows for the emulation of more complex biological processes, such as Hebbian learning, thereby enhancing the overall computational power and energy efficiency of neuromorphic systems.
The combination of synaptic transistors with neuromorphic time crystal systems is a step toward creating a highly scalable, adaptive architecture that can be tuned in real-time. By leveraging the dynamic properties of exciton-polariton condensates and the flexibility of synaptic transistors, future neuromorphic architectures could achieve a level of sophistication akin to biological neural networks, with applications ranging from pattern recognition and artificial intelligence to autonomous decision-making systems.
Comparison Between Neuromorphic Time Crystal and String Time Crystal
The comparison between neuromorphic time crystals and string time crystals reveals interesting similarities and distinctions that highlight the unique characteristics of each system. Both types of time crystals are characterized by their periodic, coherent behaviors that break time translational symmetry, but their underlying mechanisms and potential applications differ significantly.
String time crystals are conceptualized as exotic quantum states that maintain long-lived oscillations similar to the vibrations of a physical string. These systems are often studied in the context of fundamental physics, providing insights into how quantum systems can exhibit stable, periodic behavior without external periodic driving forces. String time crystals can be understood as a manifestation of time-translation symmetry breaking, wherein a system returns to its original state at regular intervals, much like the repetitive motion of a vibrating string.
On the other hand, neuromorphic time crystals leverage exciton-polariton condensates to create coherent, oscillatory networks that resemble neural architectures. These systems harness the unique properties of polaritons—hybrid quasiparticles formed through the strong coupling of photons and excitons within microcavities. Unlike string time crystals, neuromorphic time crystals are designed for practical computational purposes, utilizing their periodic behavior to perform tasks such as spiking neural network processing, optimization, and parallel computing.
One key difference between the two is their applicability. String time crystals are primarily of interest for advancing theoretical understanding in condensed matter physics, while neuromorphic time crystals have immediate implications for unconventional computing. Neuromorphic time crystals use exciton-polaritons’ rapid response times and strong nonlinearity to mimic the dynamics of biological neurons, thereby enabling energy-efficient computation that could revolutionize fields like artificial intelligence and machine learning.
Another distinction lies in their interaction mechanisms. String time crystals generally rely on intrinsic quantum properties to sustain their periodicity, whereas neuromorphic time crystals rely on the interplay between exciton-polaritons, phonon-exciton interactions, and external optical pumping to maintain their coherent oscillations. This interplay allows neuromorphic time crystals to be more readily manipulated and controlled for specific computational tasks, adding to their versatility in practical applications.
Despite these differences, the two systems share the foundational principle of using non-equilibrium conditions to sustain time-periodic behavior. Both are valuable in their respective domains—string time crystals pushing the boundaries of our understanding of quantum states, and neuromorphic time crystals providing a bridge between physics and computing technology.
References
Carraro Haddad, I., Chafatinos, D. L., Kuznetsov, A. S., Papuccio, I., Reynoso, A. A., Bruchhausen, A., Biermann, K., Santos, P. V., Usaj, G., & Fainstein, A. [2024]. Solid-state continuous time crystal with a built-in clock.
Cheng, S.-C., Jheng, S.-D., & Chen, T.-W. [2024]. Realization of photonic time crystals via spin bifurcations of exciton-polariton condensates. Optics Express, 32[13], 23524-23530.
Martins, G. P., Berman, O. L., & Gumbs, G. [2023]. Polaritonic and excitonic semiclassical time crystals based on TMDC strips in an external periodic potential. Scientific Reports, 13, 19707.
Nalitov, A. V., Sigurdsson, H., Morina, S., Krivosenko, Y. S., Iorsh, I. V., Rubo, Y. G., Kavokin, A. V., & Shelykh, I. A. [2019]. Optically trapped polariton condensates as semiclassical time crystals. Physical Review A, 99[3], 033830.
Tyszka, K., Furman, M., Mirek, R., Król, M., Opala, A., Seredyński, B., Suffczyński, J., Pacuski, W., Matuszewski, M., Szczytko, J., & Piętka, B. [2022]. Leaky integrate-and-fire mechanism in exciton-polariton condensates for photonic spiking neurons. Laser & Photonics Reviews, 17[2100660].
Opala, A., & Matuszewski, M. [2023]. Harnessing exciton-polaritons for digital computing, neuromorphic computing, and optimization. Optical Materials Express, 13[9], 2674-2680.
Sedov, E., & Kavokin, A. [2024]. Polariton lattices as binarized neuromorphic networks. Overleaf Example.
